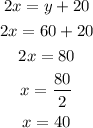Question

C. If two angles form asupplementary,then they

Answer 1

When two angles are vertically opposite then they are equal. Then, since the angles 1 and 3 are vertically opposite they are equal. Then

`<3=67^(\circ)`

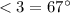
From the figure we see that we have four angles. Using the vertically opposite property we mentioned before, we have that

`\begin{gathered} x+y=x+60 \\ 2x=y+20 \end{gathered}`

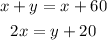
This is a system of equations, to solve it. Let's solve the first one for y.

`\begin{gathered} x+y=x+60 \\ y=x+60-x \\ y=60 \end{gathered}`

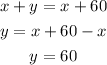
Once we have the value of y, we plug it in the second equation. Then

`\begin{gathered} 2x=y+20 \\ 2x=60+20 \\ 2x=80 \\ x=(80)/(2) \\ x=40 \end{gathered}`