You have the following function:

In order to determine the intervals, it is necessary to calculate the first derivative of the function, equal it to zero, and identify the zeros of the equation, just as follow:
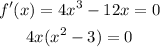
the zeros of the previous equation are:
![\begin{gathered} x_1=0 \\ x_2=\sqrt[]{3} \\ x_3=-\sqrt[]{3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/5ww8y87ddz9h19bt5nfer0qfm8wqhb7v2k.png)
Next, it is necessry if the previous values are minima or maxima. Evaluate the second derivative for the previous values of x. If the result is greater than 0, then, it is a minimum. If the result is lower than zero, it is a maximum:
![\begin{gathered} f^(\prime)^(\prime)(x)=12x^2-12 \\ f^(\prime)^(\prime)(0)=12(0)^2-12=-12<0 \\ f^(\prime)^(\prime)(\sqrt[]{3})=12(\sqrt[]{3})^2-12=24>0 \\ f^(\prime)^(\prime)(-\sqrt[]{3})=12(-\sqrt[]{3})^2-12=24>0 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/25hmf09h84hmoevln46fndb3rxjf6dtf6n.png)
Then, for x=0 there is a maximum, and for x=-√3 and x=√3 there is a minimum.
Hence, until x = -√3 the function decreases. In between x=-√3 and x=0 the function increases. In between x=0 and x=√3 the function decreases and from x=√3 the function increases.
Furthermore, it is necessary to find the inflection points. Equal the second derivative to zero and solve for x:
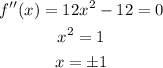
then for x=1 and x=-1 there are inflection points.
The interval where the function is concave up is:
(-∞ , -1) U (1, ∞)
The interval where the function is concave down is:
(-1,1)