Question

The average number of words in a romance novel is 64,017 and the standard deviation is 17,209. Assume the distribution is normal. Let X be the number of words in a randomly selected romance novel. Round all answers to 4 decimal places where possible.a. What is the distribution of X? X ~ N(,)b. Find the proportion of all novels that are between 69,180 and 84,668 words. c. The 95th percentile for novels is words. (Round to the nearest word)d. The middle 70% of romance novels have from words to words. (Round to the nearest word)

Answer 1

Step-by-step explanation

We are given the following parameters

`\begin{gathered} mean=\bar{x}=64,017 \\ standard\text{ deviation=}\sigma=17,209 \end{gathered}`

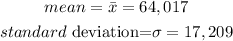
Part A

What is the distribution of X?

The distribution is

`X\text{ \textasciitilde}N=\left(\bar{x},\sigma\right)=(64017,\text{ 17209\rparen}`

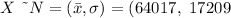
Part B

The proportion of all students that are between 69,180 and 84,668

We will follow the steps below

when x = 69,180

`z_1=\frac{x-\bar{x}}{\sigma}=(69180-64017)/(17209)=0.3000`

when x =84,668

`z_2=(84668-64017)/(17209)=1.2000`

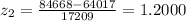
Then, we will use the statistic table to get the proportion

The proportion is 0.2670

Part C

The 95th percentile for novels is

`=1.64485362695=(x-64017)/(17209)`

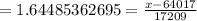
Solving for x

`\begin{gathered} x-64017=1.64485362695*17209 \\ \\ x=92323.2237 \end{gathered}`

The 95th percentile for novels is 92323 words

Part D

To get the middle 70% of romance novels are obtained as follows

we will find the value of x for which the z-score corresponds to 15% and 85%

from the table

`\begin{gathered} z_{15\text{ \%}}=-1.03643338949 \\ z_{85\text{ \%}}=1.03643338949 \end{gathered}`
`\begin{gathered} The\text{ range of words for 15\% and 85\% will be} \\ -1.03643338949=(x-64017)/(17209) \\ \\ x=46181.0761\text{ for 15\%} \\ for\text{ 85\%, we will have} \\ 1.036,433,389,49=(x-64,017)/(17,209) \\ x=81852.9238 \\ \end{gathered}`

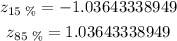
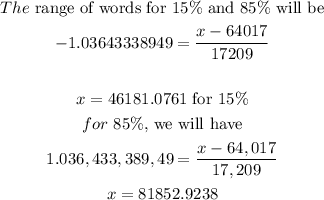
Therefore, the range of words is from 46181 words to 81853 words

267