The equation of the ellipse of center (h, k) is

Its 4 vertices are

Since the given equation is
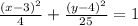
Then by comparing it with the form above
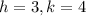
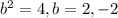
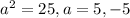
Then we can find the 4 vertices using the rule above
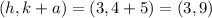
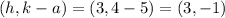
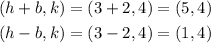
The 4 vertices are
(3, 9), (3, -1), (5, 4), (1, 4)
The center is (3, 4)