Data:
• A,( 0, 1,820,000)
,
• B,( 29, 1,200,000)
,
• Year 2 - year 1 = 2019 - 1990 = 29
a)
Based on the point A and B, the linear model is as follows:
• Slope ( ,m ,)
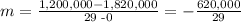
• Parameter b
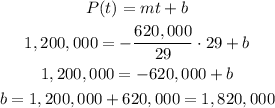
Answer a) (linear model):
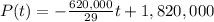
b)
2032-1990 = 42
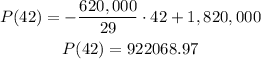
Answer of the number of violent crimes in 2032:
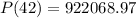
c)
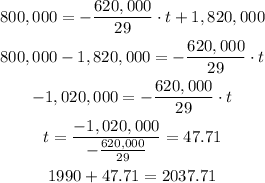
Answer of c: 2037