ANSWER

Step-by-step explanation
We want to find L's coordinates that lie along the line between the points N(14, 4) and M(2, 8) and partitions the segment in the ratio of 1 : 3.
To do this, we have to apply the section formula:
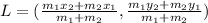
where m₁ : m₂ is the ratio
(x₁, y₁) and (x₂ : y₂) are the two coordinates of the points
Therefore, we have that the coordinates of L are:
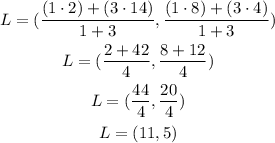
Those are the coordinates of L.