Question

An expression of the fifth degree is written with a leading coefficient of seven and a constant of six. Which expression is correctly written for these conditionsGroup of answer choices65+4+7 76−64+5 67−5+5 75+2+6

Answer 1

Given an expression of the fifth degree is written with a leading coefficient of seven and a constant of six

To Determine: Correct expression for the given polynomial

Solution:

Note that:

(i) The fifth degree means that the highest power of the polynomials is 5

i.e.

`x^5`

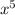
(ii) The leading coefficient is the coefficient of the highest power of the polynomial, which is the cofficient of the fifth degree. i.e.

`7x^5`

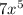
(iii) The constant is the coefficient of the variable with the power of 0. so we have

`\begin{gathered} 6x^0 \\ x^0=1 \\ 6x^0=6*1=6 \end{gathered}`

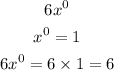
So, it should be known that the given polynomials must have the 7x⁵ as the first term and must have 6 as the last term

From the options provided, it can be concluded that the correct expression for the given condition is 7x⁵+2x+6