Step-by-step explanation
We have the simultaneous equation
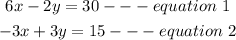
To check if (10,15) is a solution, we will have to substitute
x=10 and y=15
into both equations and check if it is true
So for equation 1
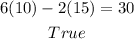
Next, for the second equation
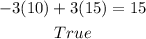
Thus,
We can conclude that (10,15) is a solution