Question

Part b: what is the apparent solution to the system of equations in the graph

Answer 1

Part B:

From the garph given, the solution to the system of equations will be the point where both lines intersect (where they meet).

The point of intersection is:

(x, y) ==> (2, 1)

Thus, we have the solution to the system of equations in the graph:

x = 2, y = 1

Part C:

Let's find the equation for both lines on the graph.

Use the slope-intercept form:

y = mx + b

where m is the slope and b is the y-intercept

For line 1:

Take the points:

(x1, y1) ==> (4, 0)

(x2, y2) ==> (0, 2)

Find the slope using the slope formula:

`\begin{gathered} m=(y2-y1)/(x2-x1) \\ \\ m=(2-0)/(0-4)=(2)/(-4)=-(1)/(2) \end{gathered}`

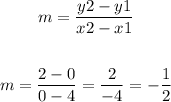
The y-intercept (b) is = 2

The slope(m) is = -½

Thus the equation of line 1 is:

y = -½x + 2

For line 2:

Take the points:

(x1, y1) ==> (0, -5)

(x2, y2) ==> (2, 1)

Find the slope:

`\begin{gathered} m=(y2-y1)/(x2-x1) \\ \\ m=(1-(-5))/(2-0)=(1+5)/(2-0)=(6)/(2)=3 \end{gathered}`

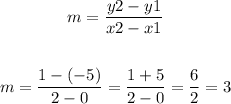
The slope(m) is 3

The y-intercept(b) is -5

Thus, the equation of line 2 is:

y = 3x - 5

Therefore, we have the system of equations:

`\begin{gathered} y=-(1)/(2)x+2.............\mleft(equation1\mright) \\ \\ y=3x-5\ldots\ldots\ldots\ldots\ldots\text{.(equation 2)} \end{gathered}`

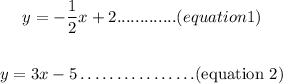
Let's solve the system.

Eliminate the equal sides and combine the equations

We have:

-½x + 2 = 3x - 5

Subtract 2 from both sides:

-½x + 2 - 2 = 3x - 5 - 2

-½x = 3x - 7

Multiply all terms by 2:

`\begin{gathered} -(1)/(2)x\ast2=3x(2)-7(2) \\ \\ -x=6x-14 \end{gathered}`

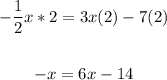
Subtract 6x from both sides:

`\begin{gathered} -x-6x=6x-6x-14 \\ \\ -7x=-14 \end{gathered}`

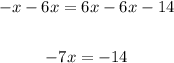
Divide both sides by -7:

`\begin{gathered} -(7x)/(-7)=(-14)/(-7) \\ \\ x=2 \end{gathered}`

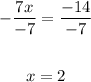
Substitute 2 for x in either of the equations.

Take equation 2:

y = 3x - 5

y = 3(2) - 5

y = 6 - 5

y = 1

Therefore, we have the solution to the system:

x = 2, y = 1

ANSWER:

x = 2, y = 1