Question

Given that events A and B are independent with P(A)=0.55 and P(B)=0.72 determine the value of P(A∣B), rounding to the nearest thousandth

Answer 1

This is a conditional probability problem

The conditional probability of A given B, denoted P(A|B), is the probability that event A has occurred in a trial of a random experiment for which it is known that event B has definitely occurred. It may be computed by means of the following formula:

To determine P(A|B)

`P(A|B)=(P(AnB))/(P(B))`
`P(A|B)=(P(AnB))/(P(B))=\frac{P(A)\text{ x P(B)}}{P(B)}`

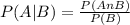
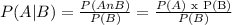
Since P(A) = 0.55 and P(B)=0.72

So,

`P(A|B)=\frac{P(A)\text{ x P(B)}}{P(B)}=\frac{0.55\text{ x 0.72}}{0.72}=0.55`

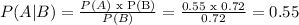
P(A|B) =0.550 (To the nearest thousandth)