Question

Consider the functionfIWhich graph is the graph of function ??

Answer 1

Step-by-step explanation:

Given:

`f(x)\text{ = }(x^2-3x)/(x-9)`

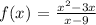
To find:

the graph that represents the function f

To determine the graph, we need to check if the expression can be simplified:

`\begin{gathered} x^2-\text{ 3x = x\lparen x - 3\rparen} \\ x^2\text{ - 9 is a differenc of two squares} \\ x^2\text{ - 9 = \lparen x - 3\rparen\lparen x + 3\rparen} \end{gathered}`
`\begin{gathered} f(x)\text{ = }\frac{x(x\text{ - 3\rparen}}{(x\text{ - 3\rparen\lparen x + 3\rparen}} \\ \\ f(x)\text{ = }\frac{x}{x\text{ + 3}} \\ \\ After\text{ simplifying, we still have a rational function} \\ We\text{ need to get the vertical asymptote} \end{gathered}`
`\begin{gathered} vertical\text{ asymptote is the value of x when the denominator is equated to zero:} \\ x\text{ + 3 = 0} \\ x\text{ = -3} \\ This\text{ means at x = -3, there is no domain and the graph will not pass through this point} \\ There\text{ will be a vertical dashed line at this point} \end{gathered}`

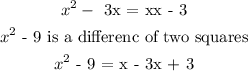
We will check the options for the graph that satisfies the above:

Only option B has a vertical dased line at x = -3. Also the graph doesn't pass t