Answer:
Equation: y - 1 = 10( x - 1)
Step-by-step explanation:
If we call x the number of days and y the number of tiles placed, then the word problem gives us two points that lie on the line we are trying to find the equation for. These points are (1,1) and (4, 31).
First, we find the slope of the line. The slope is found by dividing the difference in y coordinates by the difference in x -coordinates.
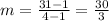
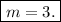
With the value of the slope in hand, we now use the point (1,1) to write the point-slope equation of the line.
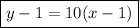
With this equation in hand, now let us find how many days it will take Lizzy to place 100 tiles. To find out we substitute y = 100 into the above equation and solve for x.
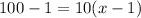
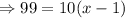
dividing both sides by 10 gives
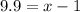
finally, adding 1 to both sides gives
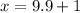

which is about 11 days.
Hence, it took lizzy exactly 10.9 days to place all the tiles.