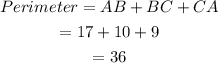Answer:
36
Step-by-step explanation:
Given the vertices of the triangle as A(-7, -4), B(1, 11), and C(-7, 5).
To be able to determine the perimeter of the triangle, we have to first find the distance between the vertices using the distance formula.
Let's determine the distance between A(-7, -4) and B(1, 11) as seen below;
![\begin{gathered} AB=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}_{}_{} \\ =\sqrt[]{\lbrack1-(-7)\rbrack^2+\lbrack11-(-4)\rbrack^2} \\ =\sqrt[]{8^2+15^2} \\ =\sqrt[]{64+225} \\ =\sqrt[]{289} \\ =17 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/tcweonwwvmhbm7fdobspamdtlky3t2pl1q.png)
Let's also determine the distance between B(1, 11) and C(-7, 5);
![\begin{gathered} BC=\sqrt[]{(-7-1)^2+(5-11)^2} \\ =\sqrt[]{(-8)^2+(-6)^2} \\ =\sqrt[]{64+36} \\ =\sqrt[]{100} \\ =10 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/l3tqmwqyvyhq4tybcpo6m0cmlalv58hnr5.png)
Let's determine the distance between C(-7, 5) and A(-7, -4);
![\begin{gathered} CA=\sqrt[]{\lbrack-7-(-7)\rbrack^2+(-4-5)} \\ =\sqrt[]{0^2+(-9)^2} \\ =\sqrt[]{81} \\ =9 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/ktmnn2phayn2ypb2aohcpt90nt8m1amaaa.png)
To find the perimeter of the triangle, we'll add the distance between each vertex together;