In this problem we know that SQ is equal to 22, we also know that the angle Q is equal to 90º and all the angles in the middle are the same and will be:

So if we separete each triangle we can find the missing angle that will be equal to 30º like this:
So now we can use the sin law to find the side SN that will be equal to the side LS so:
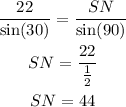
So the lenght of LS is 44