Given the function:

You can identify that its Vertex is at the Origin.
Look at the function:
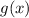
Notice that it was obtained by reflecting the first function across the x-axis and it also was shifted 2 units down.
According to the Transformation Rules for Functions:
1. If:
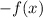
The function is reflected across the x-axis.
2. If:
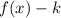
The function is shifted down "k" units.
You need to remember that the Parent Function (the simplest form) of Quadratic Equations is:
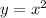
Therefore, the equation of the blue graph is:

Therefore, you can write the following equation for the second function using the rules shown before:

Hence, the answer is: Option A.