Use the Law of Conservation of Energy to find the speed of the train of the rollercoaster at the bottom of the track.
When the train is at the top of the 49.5m high track, it stores potential energy, which is given by the expression:
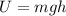
Where m is the mass of the train, g is the gravitational acceleration and h is the height of the train.
When the train travels down the track, its potential energy is released as kinetic energy, which is given by the expression:
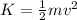
When the train reaches the bottom of the track, all of its potential energy is converted to kinetic energy, following the Law of Conservation of Energy. Then:
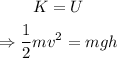
Since m is a factor on both members of the equation, it can be discarded. Then:
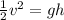
Isolate v from the equation:
![v=\sqrt[]{2gh}](https://img.qammunity.org/2023/formulas/physics/college/h5x6lakwlbvixuxzx7xrzzklyp6k7d0vvk.png)
Replace h=49.5m and g=9.81m/s^2 to find the speed of the train when it reaches the bottom of the track:
![\begin{gathered} v=\sqrt[]{2\cdot9.81(m)/(s^2)\cdot49.5m}=31.1639\ldots(m)/(s) \\ \therefore v\approx31.2(m)/(s) \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/w8ngpcuzhd4p1lj5sipehff8vj0mr1tg5g.png)
Therefore, the speed of the train of the rollercoaster at the bottom of the track is approximately 31.2 meters per second.