Answer:
8463.36 m^2
Step-by-step explanation:
Given the length(l) of the rectangle as 82m and the width(w) as 64m, we can go ahead and determine the area of the rectangle as follows;
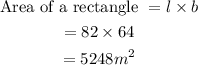
The two semi-circles are equivalent to one circle with a diameter of 64m, so the radius of the circle will be;
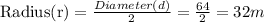
Let's go ahead and determine the area of the circle given pi as 3.14;

Therefore, the area of the training field can be determined by adding the area of the rectangle and that of the two semicircles;
