Question

35Todd forgot the first two numbers of his locker combination. The numbers can be any number 1 through 6. What istprobability that he will guess the first number correctly and the second number incorrectly?OAOB.olaroc.OD.ResetSubmit

Answer 1

A. 5/36

Step-by-step explanation:

The total number of possible number choice = 6

Only 1 out of 6 can be correct, thus:

`P(\text{he will guess the first number correctly)}=(1)/(6)`

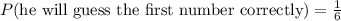
There are 5 incorrect numbers, therefore:

`P(\text{he will guess the }\sec ond\text{ number incorrectly)}=(5)/(6)`

Therefore, the probability that he will guess the first number correctly and the second number incorrectly:

`\begin{gathered} =(1)/(6)*(5)/(6) \\ =(5)/(36) \end{gathered}`

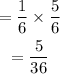
The correct choice is A.