Given the function
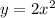
The formula to find the average rate of change of y with respect to x in [a,b]

Here, a = 1 and b = 4.
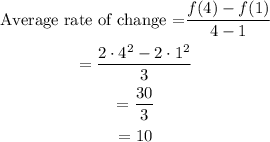
The instantaneous rate of change is the slope of the tangent line at x = 1.
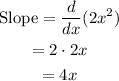
Slope at x = 1 is
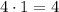
So, the instantaneous rate of change is 4.