Question

Find the volume of the composite figure.First, find the volume of the rectangularpyramid.Rectangular pyramidVolume = [?] ft³6 ftRectangular prism4 ftVolume = [] ft³Total Volume ofComposite Figure = [ ] ft³710 ft6 ft

Answer 1

Given

The composite figure,

To find the volume of the composite figure.

Step-by-step explanation:

It is given that,

The composite figure consists of a rectangular prism and a rectangular pyramid.

Then, the volume of the composite figure is given by,

`\begin{gathered} Total\text{ }volume\text{ }of\text{ }composite\text{ }figure\text{ }=Volume\text{ }of\text{ }pyramid+Volume\text{ }of\text{ }prism \\ V=V_1+V_2 \end{gathered}`

That implies,

`\begin{gathered} Volume\text{ }of\text{ }pyramid=(l* w* h)/(3) \\ V_1=(10*6*6)/(3) \\ =10*2*6 \\ =120ft^3 \end{gathered}`

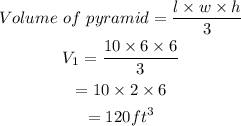
Also,

`\begin{gathered} Volume\text{ }of\text{ }prism=l* w* h \\ V_2=10*6*4 \\ V_2=240ft^3 \end{gathered}`

Therefore, the total volume of the composite figure is,

`\begin{gathered} V=120+240 \\ =360ft^3 \end{gathered}`

Hence, the volume of the composite figure is 360ft³