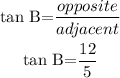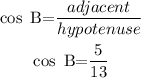Question

A right triangle has side lengths 5, 12, and 13 as shown below.Use these lengths to find tan B, sin B, and cos B.B135А12

Answer 1

The opposite side of a right angle triangle is the side facing the angle.

For angle B, the opposite side is 12;

The hypothenus side of a right angle triangle is the longest side of the triangle;

That is hypotenus equals 13;

The third side is called the adjacent and it is equal to 5;

Then, we apply the SOH CAH TOA concept to solve the problem;

`\begin{gathered} \text{tan B=}(opposite)/(adjacent) \\ \text{tan B=}(12)/(5) \end{gathered}`
`\begin{gathered} \sin \text{ B=}(opposite)/(hypotenus) \\ \sin \text{ B=}(12)/(13) \end{gathered}`
`\begin{gathered} \cos \text{ B=}(adjacent)/(hypotenuse) \\ \cos \text{ B=}(5)/(13) \end{gathered}`