Question

2C8H18(I) + 25 O2 (g) = 16 CO2(g) + 18 H2O (g)If you burned one gallon of gas (C8H18) (approximately 4000 grams) how many liters of carbon dioxide would be produced at a temp of 21.0 C and a pressure of 1.00 atm? (Answer to 3 SFs)

Answer 1

The reaction is balanced, so we can proceed with the calculations. To solve the question we will follow the following steps:

1. We calculate the moles of C8H18, dividing the mass of the fuel by its molar mass. The molar mass of C8H18 is: 114.23g/mol.

2. By stoichiometry we find the moles of CO2 that are produced from the moles of C8H18 found.

3. We find the volume of CO2 formed using the ideal gas law which tells us:

`PV=nRT`
`V=(nRT)/(P)`

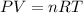
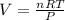
Where,

P is the pressure of the gas in atm, 1.00 atm

T is the temperature of the gas in Kelvin, 21.0°C=294.15K

R is a constant, 0.08206atm.L/mol.K

n is the number of moles of the gas

V is the volume of the gas in liters

Let's continue with the calculations.

1. Moles of C8H18

`molC_8H_(18)=givengC_8H_(18)*(1molC_8H_(18))/(MolarMass,gC_8H_(18))`
`molC_8H_(18)=4000gC_8H_(18)*(1molC_8H_(18))/(114.23gC_8H_(18))=35.0molC_8H_(18)`

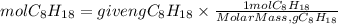
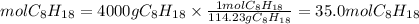
2. Moles of CO2

The ratio CO2 to C8H18 is 16/2, so the moles of CO2 formed will be:

`molCO_2=givenmolC_8H_(18)*(16molCO_2)/(2molC_8H_(18))`
`molCO_2=35.0molC_8H_(18)*(16molCO_2)/(2molC_8H_(18))=280molCO_2`

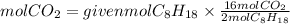
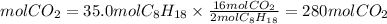
3. Volume of CO2

We replace the known data in the gas ideal law:

`V=(nRT)/(P)`
`V=(280mol*0.08206(atm.L)/(mol.K)*294.15K)/(1.00atm)=6761.9L=6.76*10^3L`

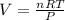
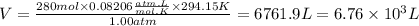
Answer: Would be produced 6.76x10^3 liters