Question

Suppose that two objects attract each other with a gravitational force of 16 units. If the mass of object 1 was doubled, and if the distance between the objects was tripled, then what would be the new force of attraction between the two objects?

Answer 1

Given:

The gravitational force between two objects is,

`16\text{ units}`

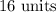
The mass of object 1 was doubled.

The distance between the objects was tripled.

To find:

The new force of attraction between the two objects

Step-by-step explanation:

The force of attraction between two objects at a distance 'r' is,

`F=(Gm_1m_2)/(r^2)`

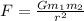
According to the question,

`(Gm_1m_2)/(r^2)=16`

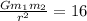
The new force of attraction is,

`\begin{gathered} F^(\prime)=(G*2m_1* m_2)/((3r)^2) \\ =(2Gm_1m_2)/(9r^2) \\ =(2)/(9)F \\ =(2)/(9)*16\text{ units} \\ =(32)/(9)\text{ units} \end{gathered}`

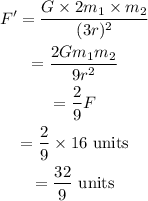
Hence, the new force of attraction is 32/9 units.