Answer:
The standard error is 0.0157.
The 90% confidence interval for the proportion of U.S. adults who are dissatisfied with the education students receive in kindergarten through grade 12 is (0.5042, 0.5558). This means that we are 90% sure that the true proportion of U.S. adults who are dissatisfied with the education students receive in kindergarten through grade 12 is between 0.5042 and 0.5558.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
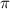 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
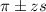
In which
z is the zscore that has a pvalue of
 .
.
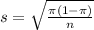 is the standard error.
is the standard error.
In a Gallup survey of 1,012 randomly selected U.S. adults (age 18 and over), 53% said that they were dissatisfied with the quality of education students receive in kindergarten through grade 12.
This means that
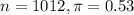
The standard error is:
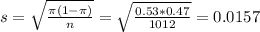
The standard error is 0.0157.
90% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
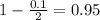 , so
, so
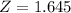 .
.
The lower limit of this interval is:

The upper limit of this interval is:
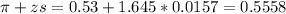
The 90% confidence interval for the proportion of U.S. adults who are dissatisfied with the education students receive in kindergarten through grade 12 is (0.5042, 0.5558). This means that we are 90% sure that the true proportion of U.S. adults who are dissatisfied with the education students receive in kindergarten through grade 12 is between 0.5042 and 0.5558.