Population in 2014=23.2 million
Population in 2018=26.8 million
Population in 2024= ?
The population increase is linear, therefore:
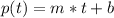
Where t, time since 2005 and p is the population.
We know two points:
P1= (t, p) = (2014, 23.2)
P2=(t , p)=(2018, 26.8)
But the time is since 2005, then:
2014-2005=9
2018-2005= 13
The points are:
P1= (t1, p1) = (9, 23.2)
P2=(t2 , p2)=(13, 26.8)
The slope is going to be:
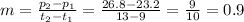
Replacing any point in the equation to find b:

The equation is:
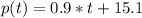
Where 15.1 millions is the population in 2005.
Finally, the population in 2024 will be:

Answer: The population of Nevada in 2024 is: 32.2 millions.