Question

I need help with this study guide so I can use it to study for a test.

Answer 1

1. Colinear points are the ones that are in the same line.

In this example, K is colinear to J and L (referring to line a).

It can be N and M, referring to line c.

2. As the line has the points H and K included, it can be named HK.

3. The intersection of a line and a plane, assuming that the line is not included in the plane, is a point. In this case, the intersection of line c and plane R is point K.

4. A point is coplanar to a plane or another point when it belongs to the plane.

In this case the point M is not coplanar to the plane R (it is outside of the plane).

5. Each face of the prism is a plane and it is can be defined by 3 points. So in this case we have 5 planes (four faces and one base, that is W).

6. We can assign a name for a plane with 3 points that belong to that plane. A possible name is ABC.

7. The intersection of plane W and ADE is the line that goes through the points A and D. We can call this line with the name AD.

8. E, C or D are no colinear to A and B. They do not belong to the line that goes through the points A and B (or the line AB).

9. DF is the sum of DE and EF, so we have:

`\begin{gathered} \bar{DF}=\bar{DE}+\bar{EF} \\ \bar{DF}=(7x+1)+(4x-3)=42 \\ 11x-2=42 \\ 11x=42+2=44 \\ x=(44)/(11)=4 \\ \\ \bar{DE}=7x+1=7\cdot4+1=28+1=29 \end{gathered}`

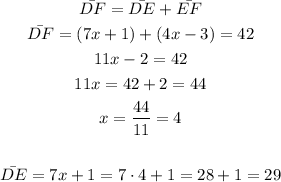
DE = 29

10. We know that:

`\begin{gathered} JK+KL=JL \\ (5x-8)+(7x-12)=10x-2 \\ 12x-20=10x-2 \\ 12x-10x=-2+20 \\ 2x=18 \\ x=(18)/(2) \\ x=9 \\ \\ KL=7x-12=7\cdot9-12=63-12=51 \end{gathered}`

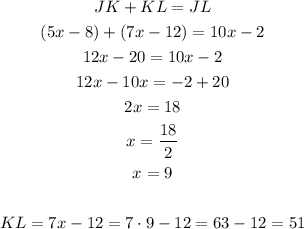
11. If S is the midpoint of RT, then RS=ST.

We would have:

`\begin{gathered} RS=ST \\ 5x+17=8x-31 \\ 5x-8x=-31-17 \\ -3x=-48 \\ x=(-48)/(-3)=16 \\ \\ ST=8x-31=8(16)-31=128-31=97 \end{gathered}`

12. If y bisects AC, then AB=BC. Then we have:

`\begin{gathered} AB=BC \\ 4-5x=2x+25 \\ -5x-2x=25-4 \\ -7x=21 \\ x=(21)/(-7)=-3 \\ \\ AC=AB+BC \\ AC=(4-5x)+(2x+25)=(4-5\cdot(-3))+(2\cdot(-3)+25) \\ AC=(4+15)+(-6+25)=19+19 \\ AC=38 \end{gathered}`

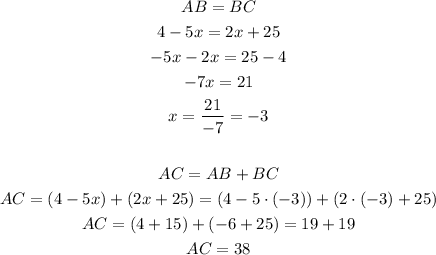
13. AB is half the value of AC, so we have:

`\begin{gathered} 2\cdot AB=AC \\ 2(3x+4)=11x-17 \\ 6x+8=11x-17 \\ 6x-11x=-17-8 \\ -5x=-25 \\ x=5 \end{gathered}`

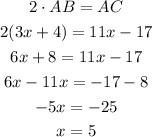
Then, we can calculate CD = AC

`CD=AC=11x-17=11(5)-17=55-17=38`

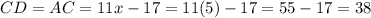
We can define DE as DE = CE - CD. We can calculate it as:

`DE=CE-CD=49-38=11`

DE = 11