Step-by-step explanation
To find the length of the hypotenuse using the right triangle ratio
Since the angles are in the ratio
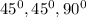
So the side ratio will be in the ratio
![1\colon1\colon\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/41tpjsiy7t1v94h6ct8dqgh148zi7wqje2.png)
Thus,
If x = 15 and the hypotenuse is y
Then
![\begin{gathered} 45^0\colon45\colon90^0 \\ 15\colon x\colon y \\ 1\colon1\colon\sqrt[]{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/4nq0fqwmgfek9lb8iendgq64s8jz5h3a8a.png)
Thus, from the above relationship
![\begin{gathered} x=15 \\ y=15*\sqrt[]{2}=15\sqrt[]{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/zd6py93gurt0l9llk8cgtmuomxe8cnl95d.png)
Therefore, the hypotenuse is
![15\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/sd6bdju1b2xzhdqbnemi68lz97aknlbhh6.png)