Question

Consider function f, where 8 is a real number.J(=) = tan(Br)Complete the statement describing the transformations to function fas the value of B is changed.As the value of B increases, the period of the functionV, and the frequency of the functionWhen the value of B is negative, the graph of the function (reflects over the y-axis

Answer 1

As the value of B increases, the period of the function decreases, and the frequency of the function increases. When the value of B is negative, the graph of the function reflects over the x-axis

Explanation:

The period of a trigonometric function is given by the following equation:

`\begin{gathered} T=(\pi)/(k) \\ \\ \text{ let k be the constant number that is the coefficient of the angle x} \end{gathered}`

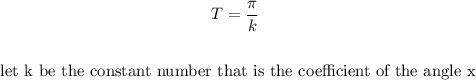
In this case, k is equal to B, if B increases then the period decreases, and since the frequency is inversely proportional to the period it would increase.

If the value of B is negative we would have the following:

`f(x)=\tan(-Bx)=-\tan(Bx)`

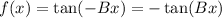
Therefore, there is a reflection on the x-axis.

As the value of B increases, the period of the function decreases, and the frequency of the function increases. When the value of B is negative, the graph of the function reflects over the x-axis