we have the sequence
15, -85, -185, -285, ...
so
a1=15 first term
a2=-85
a3=-185
a4=-285
Find out the difference between consecutive terms
a2-a1=-85-(15)=-100
a3-a2=-185-(-85)=-185+85=-100
a4-a3=-285-(-185)=-285+185=-100
The difference is a constant
that means ----> this is an arithmetic sequence
where
the common difference d=-100
The formula to calculate the a_n term is given by
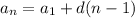
To find out the 30th term
we have
d=-100
n=30
a1=15
substitute
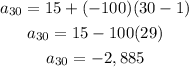
The 30th term is -2,885