Given that she bought a total of 10 items ( pants and t-shirts) each pair of pants cost $53, each shirt is $27. She spent $374, we have two linear equations as follows:
Let x be the number of pants and let y be the number of t-shirts, then:
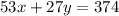
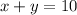
In order to find how many and shirts she bought, we have to solve this linear system of two equations and two variables:
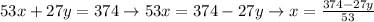
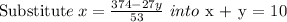
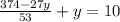
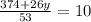
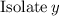
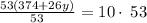
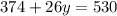
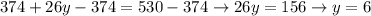
then:

So, she bought 4 pants and 6 t-shirts.