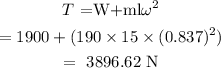Question

(Figure 1)An amusement park ride consists of airplane-shaped cars attached to steel rods. Each rod has length 15 m. Assume the rod's weight is irrelevant.The cart has a weight of 1900 NWhen the ride is operating, it has a maximum angular speed of ω=8.0rev/min . How much tension is on the rod?

Answer 1

Given:

The length of the rod is l = 15 m

The weight of the cart is W = 1900 N

The angular speed is

`\begin{gathered} \omega\text{ = 8 rev/min} \\ =8*(2\pi)/(60) \\ =\text{ 0.837 rad/s} \end{gathered}`

To find the tension in the rod.

Step-by-step explanation:

First, we need to calculate the mass of the cart.

The mass can be calculated as

`\begin{gathered} m=(W)/(g) \\ =(1900)/(10) \\ =\text{ 190 kg} \end{gathered}`

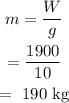
The tension in the rod can be calculated as

`\begin{gathered} T\text{ =W+ml}\omega^2 \\ =1900+(190*15*(0.837)^2) \\ =\text{ 3896.62 N} \end{gathered}`