1 Answer

Phanto · Answer 1 · 2023-01-08T02:18:51+0000

Given: A figure with a diameter of 16 in and a slant height of 17 in.

Required: To name the figure and find out the surface area of the given figure.

Explanation: The given figure is a cone. The surface area of a cone with radius 'r' and slant height 'l' is given by the formula-

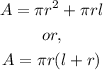
$\begin{gathered} A=\pi r^2+\pi rl \\ or, \\ A=\pi r(l+r) \end{gathered}$

Given,

$\begin{gathered} r=(16)/(2) \\ r=8\text{ in} \\ l=17\text{ in} \end{gathered}$

Hence, the surface area of the cone is

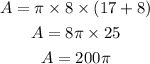
$\begin{gathered} A=\pi*8*(17+8) \\ A=8\pi*25 \\ A=200\pi \end{gathered}$

Or,

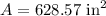
$A=628.57\text{ in}^2$

Final Answer: The given figure is a cone with a surface area of 628.57 square inches.

Please log in or register to add a comment.