Given:
Altitude of plane = 11,000 feet
Angle of depression = 7 degrees
Let's find the how far away from the runway should the pilot begin to descend.
Let's first draw a figure that represents this situation:
Here, we are to solve for x.
Since the pilot is to make a descent to the runway, we are asked to find the horizontal distance fro
To solve for x, apply the trigonometric ratio formula for tan.
Thus, we have:
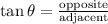
WHere:
Opposite side is the side opposite the given angle = x
Adjacent side is the side adjacent to the given angle = 11000
θ = 7 degrees
Thus, we have:
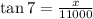
Let's solve for x:
Multiply both sides by 11000
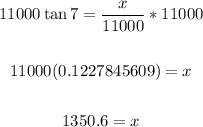
x = 1350.6 ft
Therefore, the pilot should begin the descent at a distance of 1350.6 ft from the runway.
ANSWER: