In point reflection, the distance from the preimage to the point of reflection is the same as the distance from the point of reflection to the image.
Hence, this means that if the preimage coordinates are
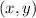
and the image coordinates are
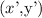
and are reflected over a point
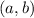
It thus follows that
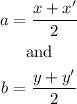
From the question, the preimage is given as
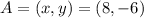
and it is reflected over the point
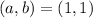
Applying the formula above, we can calculate the coordinates of the image B