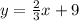In order to find the equation, let's use the slope-intercept form:

Where m is the slope and b is the y-intercept.
Parallel lines have the same slope, so if the slope of the given line is 2/3, the slope of the wanted parallel line is m = 2/3.
Now, to find the value of b, let's use the point (-9, 3) in the equation:
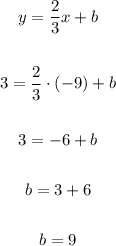
So the wanted equation is: