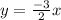Question

14. Write an equation in slope-intercept form for the line that passes through

(-2, 3) and is parallel to the line with equation 3x + 2y = 6.

Answer 1

`y=(-3)/(2) x`

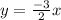
Explanation:

`3x+2y=6`

`P(-2,3)`

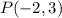
Rewrite the first equation in slope-intercept form:

`2y=-3x+6`

`y=(-3)/(2) x+3`

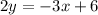
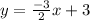
The slope-intercept form is the following:

`y=mx+b`

m = slope

parallel lines have the same slope, therefore we know that our new line will have a slope (-3/2).

Now write the line in the point-slope form:

`(y-y_1)=m(x-x_1)`

`y-3=(-3)/(2) (x+2)`

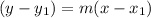
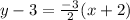
Now we need to re-write into slope-intercept form. Distribute -3/2:

`y-3=(-3)/(2) x-3`

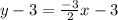
Add 3 to both sides:

`y=(-3)/(2) x`