Question

(-3,2) and (-11,-1) type an equation

Answer 1

The equation of the line that passes through the two points is;

`y=(3)/(8)x+(25)/(8)`

Step-by-step explanation:

Given the two points;

`(-3,2)\text{ and }(-11,-1)`

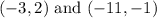
Firstly, let us find the slope of the line;

`m=(\Delta y)/(\Delta x)=(y_2-y_1)/(x_2-x_1)`

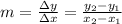
Substituting the given points, we have;

`\begin{gathered} m=(-1-2)/(-11-(-3))=(-3)/(-8) \\ m=(3)/(8) \end{gathered}`

Then to get the equation let us substitute the slope and the first point into the point-slope form of equation of line;

`y-y_1=m(x-x_1)`

Substituting and simplifying;

`\begin{gathered} y-2=(3)/(8)(x-(-3)) \\ y-2=(3)/(8)(x+3) \\ y-2=(3)/(8)x+(9)/(8) \\ y=(3)/(8)x+(9)/(8)+2 \\ y=(3)/(8)x+(25)/(8) \end{gathered}`

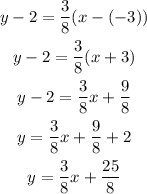
Therefore, the equation of the line that passes through the two points is;

`y=(3)/(8)x+(25)/(8)`