Question

Peter has half of his investments in stock paying a 6% dividend and the other half in a stock paying 14 % interest. If his total annualinterest is $500, how much does he have invested?

Answer 1

Assume that Peter will invest $x

Then he will invest 1/2 x with a rate of 6% and 1/2 x with a rate of 14%

Since the rule of the interest is

`I=\text{PRT}`

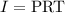
P is the amount of investment

R is the rate in decimal

T is the time

For the first account

`\begin{gathered} P=(1)/(2)x \\ R=(6)/(100)=0.06 \\ T=1 \end{gathered}`

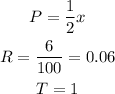
For the second account

`\begin{gathered} P=(1)/(2)x \\ R=(14)/(100)=0.14 \\ T=1 \end{gathered}`

Then the interest of each account is

`\begin{gathered} I_1=((1)/(2)x)(0.06)(1) \\ I_1=0.03x \end{gathered}`
`\begin{gathered} I_2=((1)/(2)x)(0.14)(1) \\ I_2=0.07x \end{gathered}`

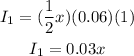
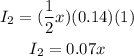
Since the total interest is $500, then add I1 and I2, equate the sum by 500

`\begin{gathered} I_1+I_2=500 \\ 0.03x+0.07x=500 \\ 0.10x=500 \end{gathered}`

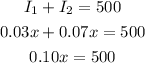
Divide each side by 0.10

`\begin{gathered} (0.10x)/(0.10)=(500)/(0.10) \\ x=5000 \end{gathered}`

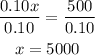
Then he invested in each account 5000/2 = $2500

He invested a total of $5000