ANSWER
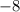
Step-by-step explanation
To find the slope of the function at the given point, we have to apply the formula:
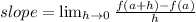
where a = x-coordinate of the point.
From the question, a = 2.
Therefore, the slope of the function at x = 2 is:
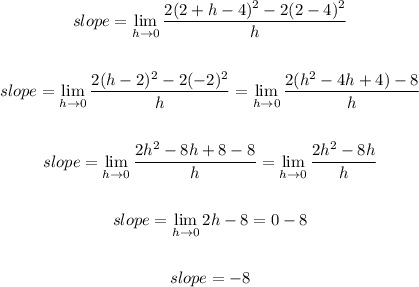
That is the slope at the given point.