Question

Next PagePage 6 of 11Question 6 (3 points)A patient is injected with 10 mg of a radioactive isotope called Iodine 131. The half-life of lodine 131 is eight days.• Determine the exponential equation of this scenario.• How many milligrams of Iodine-131 is left in the body after 4 days? Roundyour answer to the nearest tenth of a milligram.. At what point of time will the amount remaining in the patients system be lessthan 0.5 mg? Round answer to the nearest day,

Answer 1

we have that

In this problem, we have an equation of the form

`y=a((1)/(2))^{((x)/(8))}`

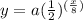
where

y ------> milligrams of Iodine-131 left in the body

x -----> number of days

a ----> initial value

a=10 mg

substitute

`y=10((1)/(2))^{((x)/(8))}`

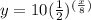
Part 2

For x=4 days

`\begin{gathered} y=10((1)/(2))^{((4)/(8))} \\ y=7.1\text{ mg} \end{gathered}`

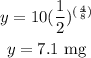
Part 3

y < 0.5 mg

we have the inequality

`10((1)/(2))^{((x)/(8))}<0.5`

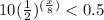
solve the inequality

`\begin{gathered} ((1)/(2))^{((x)/(8))}<(1)/(20) \\ ((x)/(8))\cdot\log (0.5)<\log ((1)/(20)) \\ x<34.57 \end{gathered}`

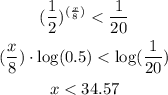
therefore

the number of days must be less than 35 days