SOLUTION
The solution to the questions is obtained from the interpretation of the graph.
Consider the image of the graph given
From the diagram above, the cost to get into a red cab is when the miles is at zero which is the y-intercept
From the graph above, the cost of getting into a red cab is
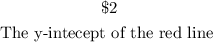
Hence
1). The cost to get into a red cab is $ 2
For the red cab, we use the red line
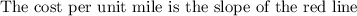
The slope of the red line is obtained by

Hence
2). The cost per mile for a red cab is $2 per mile
The equation of the line in slope and intercept form is given by
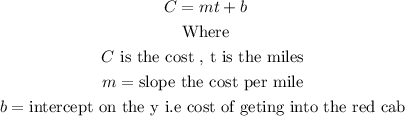
Since
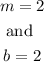
Then, the required equation is

Therefore
3). The equation in slope-intercept form that relates the cost to the miles travelled for a red carb is C = 2t + 2