ANSWER
a6 = 108864
Step-by-step explanation
First, we have to find the rule to obtain the nth term of the sequence. For that, we have to decide if this sequence is an arithmetic sequence or a geometric sequence. Note that between the first and second term there's a difference of 70, but between the second and third terms the difference is 420. Therefore it can't be an arithmetic sequence.
Let's assume it is a geometric sequence. The formula for the nth term is:
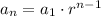
We have a1 = 14 and using the second term we can find r. a2 = 84:
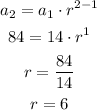
The general formula for the nth term of this sequence is:
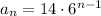
To be sure, let's check if it works for the third term:
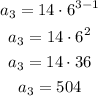
Now, to find the 6th term, we just have to use this formula, with n = 6:
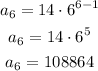
Hence, the 6th term of this sequence is 108864.