Question

TRIGONOMETRY What is cos(30 degrees)?

Answer 1

We need to find the value of cos(30°).

Now, in trigonometric the function of cosine is defined as the ratio of the adjacent side to the hypotenuse:

`\text{cos}\theta=\frac{adjacent\text{ side}}{\text{hypotenuse}}`

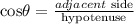
If the given angle of the right triangle is 30 degrees.

We have the next situation:

Now, using the values on a unit circle, we have the next values:

Where the largest side always represents the hypotenuse and the opposite side is the opposite side to the given angle.

Hence:

Hypotenuse = 2

Opposite side = 1

We need to find the adjacent side using the Pythagorean Theorem, which is given by:

Hypotenuse²= opposite side² + adjancet side²

Solve for the adjacent side:

adjacent side =√(Hypotenuse² - opposite side²)

Replacing:

adjacent side =√(2² - 1²)

adjacent side =√3

Finally, replace using the cosine function:

cos 30 = adjacent side / hypotenuse

Hence, the result is:

`\cos 30=\frac{\sqrt[]{3}}{2}`