For this exercise you need to remember the following Transformation rules for for a function f(x):
1) If:
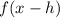
Then the function is shifted right "h" units.
2) If:
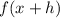
Then the function is shifted left "h" units.
In this case you have this parent function:

Which is a line that passes through the point (0,0) or "The origin".
You know that the function g(x) is a transformation of f(x), and this is:
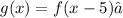
So you can identify that the transformation has the form:
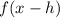
Where:
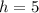
Therefore, the graph of g(x) is the graph of f(x) but shifted right 5 units.
Then, the graph of the function g(x) is: