Question

I will send a picture of the equation because it won't make sense if I type it here.

Answer 1

To find the answer, we will need to replace the population P by 50,000 and solve the initial equation for t because t is the number of years after 2012.

So, we get:

`50,000=25,000e^(0.03t)`

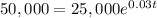
Now, we need to remember some properties of the logarithms:

`\ln e^a=a`

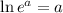
Then, we can solve for t as:

`\begin{gathered} 50,000=25,000e^(0.03t) \\ (50,000)/(25,000)=(25,000e^(0.03t))/(25,000) \\ \\ 2=e^(0.03t) \end{gathered}`

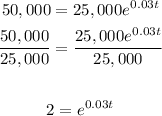
So, using the property, we get:

`\begin{gathered} \ln 2=\ln e^(0.03t) \\ \ln 2=0.03t \end{gathered}`

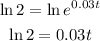
Finally, dividing by 0.03 into both sides, we get that the number of years after 2012 that the population will be 50,000 is:

`\begin{gathered} (\ln 2)/(0.03)=(0.03t)/(0.03) \\ (\ln 2)/(0.03)=t \end{gathered}`

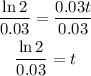
Answer: t = ln2/0.03