Given:
The object's height is h = 3 cm
The object's distance from the concave mirror is u = -20 cm
The radius of curvature is R = - 30 cm
Required:
(a) Image's distance from the mirror
(b) Image's height
Step-by-step explanation:
The focal length of the mirror can be calculated as
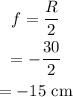
(a) The image's distance can be calculated using the mirror formula as
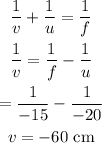
(b) The image's height can be calculated as
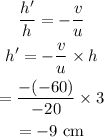
Final Answer:
(a) Image's distance from the mirror is v = -60 cm
(b) Image's height is h' = -9 cm