Question

Use elimination to solve eachsystem of equations3x + y = 26x + 2y = 4

Answer 1

Given the system of equations:

`\begin{gathered} 3x+y=2 \\ 6x+2y=4 \end{gathered}`

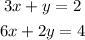
We will use the elimination method to solve the system of equations:

So, we will multiply the first equation by (-2) to eliminate y:

`\begin{gathered} 3x+y=2\rightarrow(*-2) \\ 6x+2y=4 \\ ========= \\ -6x-2y=-4 \\ 6x+2y=4 \end{gathered}`

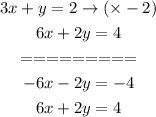
Add the equations:

`\begin{gathered} (-6x+6x)+(-2y+2y)=-4+4 \\ 0=0 \end{gathered}`

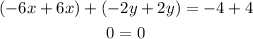
The last result means that the system is consistent dependent

So, there are the infinite solutions because the equations represent the same line

So, the answer will be an infinite solution