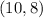Let be "x" the number of nickels Evelyn has and "y" the number of pennies she has.
According to the information given in the exercise, she has a maximum of 20 coins. Then, you can set up the following inequality to represent it:
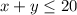
Because "a maximum of 20 coins" indicates that the total number of coins is less than or equal to 20.
You also know that that money is worth a minimum of $0.40. Then, you can set up this inequality:
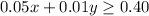
Since the first inequality is:
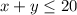
You need to solve for "y" in order to rewrite it:
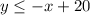
Knowing that the second inequality is:
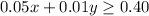
You can solve for "y" in order to rewrite it:
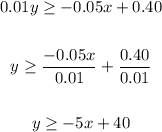
Therefore, the System of Inequalities is:
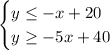
The Slope-Intercept Form of the equation of a line is:

Where "m" is the slope and "b" is the y-intercept.
- Notice that the first line of the system is:

You can identify that:
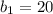
You can find the x-intercept by substituting the following value of "y" into the equation and solving for "x":

Then, you get:
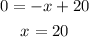
Therefore, you know that the first line passes through these points:
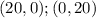
- Since the second line is:
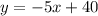
You can determine that:
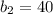
To find the x-intercept, apply the same procedure used for the first line:
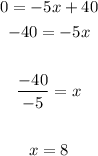
Then, the line passes through these points:
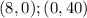
- Notice that the symbol of the first inequality is:
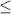
That indicates that the first line is solid and the shaded region must be below the line.
- The symbol of the second inequality is:
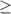
This indicates that the line is solid and the shaded region must be above the line.
Knowing the explained above, you can graph the System of Inequalities:
By definition, the solution of the System of Inequalities is the intersection region.
Then, in order to determine one possible solution, you can choose a point in the intersection region. This can be (the solution contains this point):
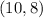
Therefore, answers are:
-Inequality 1:
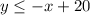
- Inequality 2:
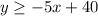
- Graph:
- The solution contains this point: