Question

Identify two similar triangles in the figure below, and complete the explanation of why they are similar. Then find AB. B А C 21 D ZA = (select) and ZABD = (select), so ABD - ACB by the (select)y Triangle Similarity Theorem. AB =

Answer 1

∠A ≅ ∠A and ∠ABD ≅ ∠ACB, so ΔABD ≅ ΔACB by the AA (Angle - Angle) Triangle Similarity Theorem.

AB = 10

Step-by-step explanation:

An angle is congruent to itself, so ∠A ≅ ∠A

On the other hand, taking into account the representation of the angles, we can say that: ∠ABD ≅ ∠ACB

Then, the triangles ABD and ACB are congruent by the AA (Angle-Angle) triangle similarity theorem because we have two congruent angles:

∠A ≅ ∠A

∠ABD ≅ ∠ACB

Now, if two triangles are similar their corresponding sides are proportional.

So, we can formulate the following equation:

`(AB)/(AC)=(AD)/(AB)`

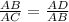
Then, replacing AC by (21 + 4), AD by 4, and solving for AB, we get:

`\begin{gathered} (AB)/(21+4)=(4)/(AB) \\ AB* AB=4(21+4) \\ AB^2=4(25) \\ AB^2=100 \\ AB=\sqrt[]{100} \\ AB=10 \end{gathered}`

Therefore, the answers are:

∠A ≅ ∠A and ∠ABD ≅ ∠ACB, so ΔABD ≅ ΔACB by the AA (Angle - Angle) Triangle Similarity Theorem.

AB = 10