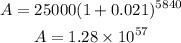Question

A new car cost $23,427. It's value decreases by 34% each year.

Answer 1

a) 25000(1+0.021)^t

b) 1.28 x 10^(57)

Step-by-step explanation:

To calculate the balance after t days, we can use the following equation:

`A=P(1+i)^t`

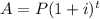
Where P is the initial investment, i is the interest rate daily and t is the number of days.

Therefore, the model for this situation is:

`A=25000(1+0.021)^t`

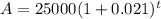
Then, if 16 years is equivalent to 5840 days, the balance after 16 years is equal to:

`\begin{gathered} A=25000(1+0.021)^(5840) \\ A=1.28*10^(57) \end{gathered}`